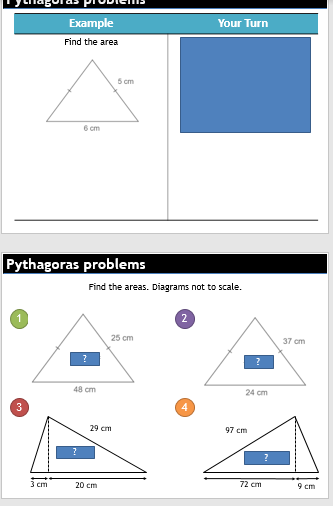
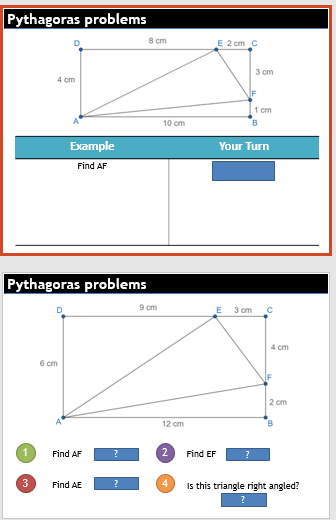
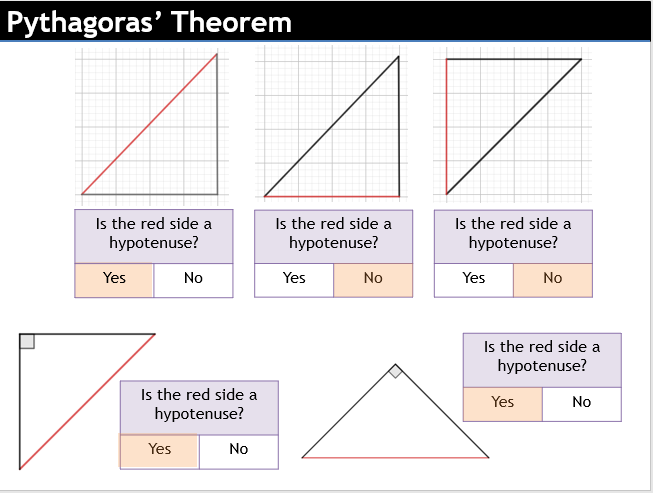
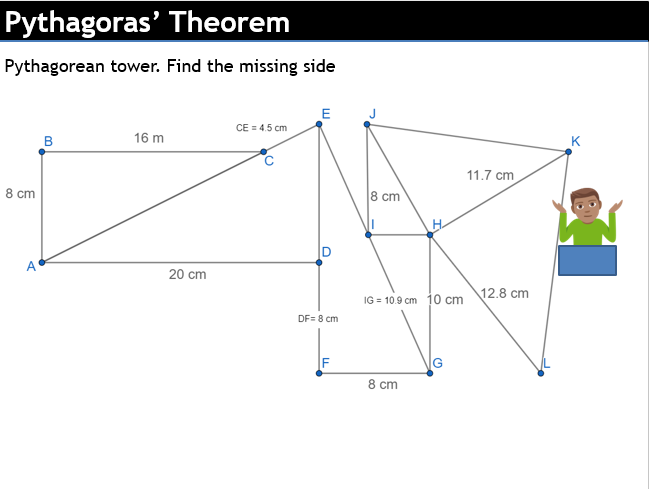
So … I can’t claim that I’ve done much original work here.
Most of this is stolen from Jo Morgan’s indices in depth presentation. Sorry Jo!
Normally I make a lot of stuff from scratch, but Jo’s work here is so good that I can’t really improve on it. If you get to see Jo do an ‘in depth’ presentation, go. The time and effort behind them is phenomenal.
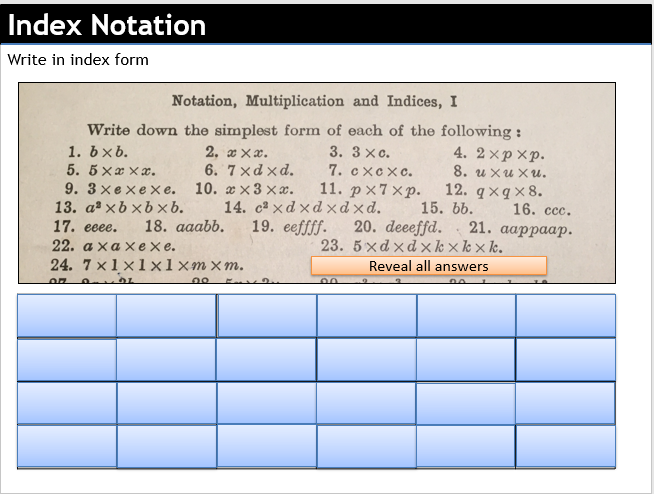
PowerPoint contains a section on writing things in index and expanded form.

As well as some questions people might find a bit old fashioned. Also the first time I’ve put a ‘reveal all’ button on a page. Can’t believe I’ve not thought to do that before.
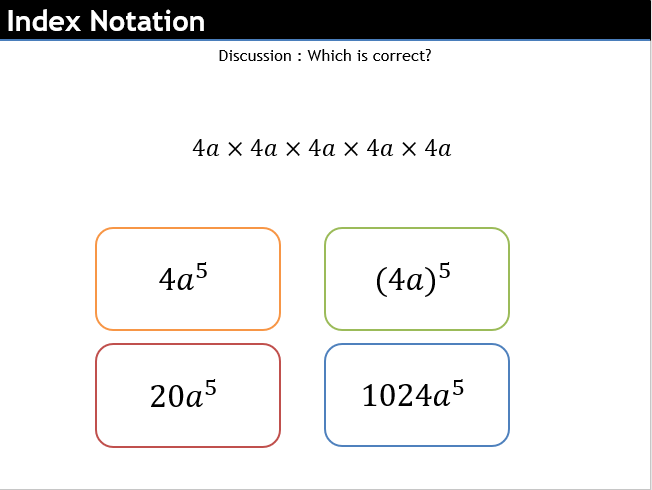
I like these little discussion slides. I think they’re fun.
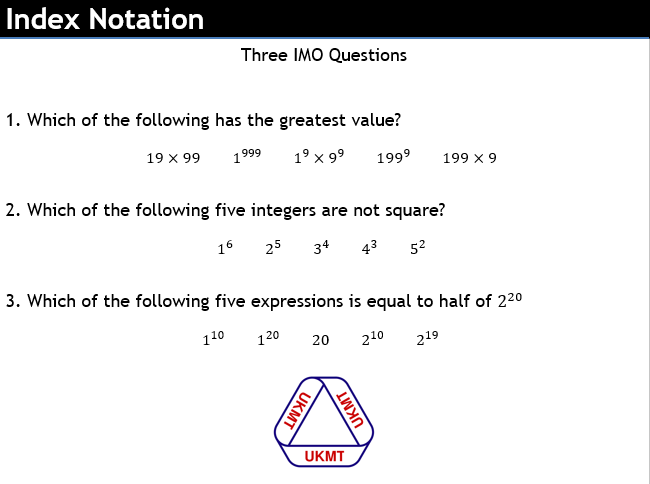
Some UKMT questions, which I stole from this book. It’s a lovely little book.
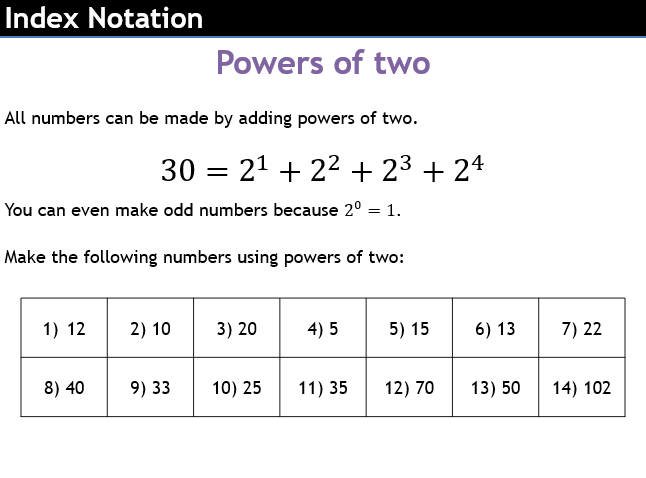
I also added a little problem solvey bit where we talk about powers of two and binary.
Multiplying to come next (it’s basically copied and pasted from Jo again. Sorry Jo!)