TickTockMaths

Completing the square

Sometimes you look back at your old work and feel it was written by another person. I went to teach completing the square this week and I couldn’t understand my own resource. It was all over the place. I’d clearly thought about activity first and the learning journey second. It was all messed up. I introduced ax^2 + bx + c waaaay to quickly.

Well now it’s a lot better (in my opinion).

There’s an example problem pair with a simple example and two exercises on doing the simple stuff before we go anywhere near anything harder.

THEN we move on to doing harder ones, and finally turning points.

There’s also a matching worksheet with all the questions on.

I’ve deleted the old resource.

-Rich

Writing in standard form

Get the PowerPoint here

Please tell me if you can’t download from the above link. Hopefully you can also see a preview of the PowerPoint above. (Clicking the ‘book’ icon should open a menu that will allow you to download a copy for yourself)

Been meaning to update this one for a while. The old ones were not good.

Firstly, I’ve gone for FRESH NEW LOOK

I am trying to do some more etymology stuff this year, so I’ve got a dedicated bit talking about it. Mainly, I’ve got rid of the big picture and the learning objective (which was usually just the title anyway). I’ve also tried to minimise my use of dark bits as you’ll see.

These animate in. I think it’s really interesting to be specific. Talk about what is and isn’t standard form. In my early teaching career I definitely rushed through stuff and it makes me cringe a bit.

Obviously before you do this, you need to tell them what standard form is!

Then we do an example problem pair and then this

I love moving between different forms and having to think backwards. Blocking out the tables is always good. I also like getting students to write out the full expanded form.

I do the same for negative indices and then I added this

I think it’s important that we don’t lose the point of standard form, which is easy comparison of numbers and scale. All that stuff like Scale of the Universe (https://htwins.net/scale2/) which makes this topic cool!

Please hit me up on Twitter if you have feedback or comments!

Things I Think I Think … 1

I know I haven’t blogged for ages. Sometimes it’s a bit daunting doing a proper post with loads of resources etc. So I’m just going to jot some thoughts down.

1. Craftsmanship Videos

I’m a bit obsessed with videos like this at the moment.

There’s so much wonderful about them, but I’ve come to have a realisation about doing things in a very deliberate way.

The woman takes care and attention over every part of her process. Nothing is done in a thoughtless way. She is not doing things automatically. It’s kinda how I aim for myself to behave as a teacher (not saying I always hit that height) and how I want my students to behave. Taking care. Thinking. Not just doing an automatic process.

I’ve noticed that sometimes pupils are particularly prone to slipping into automatic mode in a test. This year I’ve really tried to point out and develop self-talk but there’s so much more I need to look at there …

2. Old Resource Issues

Talking of a lack of care, I’ve been looking at some of my resources that are a bit old.. and I’m quite embarrassed. I really need to do a full audit of the site to remove the junk (more on that idea later) . Let’s talk about a particularly egregious one. This is my Solving Equations (with brackets) PowerPoint. It’s terrible.

First, let’s look at this example-problem pair

There’s so many things wrong with it. Both questions can be divided nicely by the factor outside the bracket. What happens when this isn’t the case? Clearly not a huge amount of thought has been put into these examples

And then you have the problem set

I have spent 100x more time trying to design some sort of fun thing here than on the actual questions. They’re terrible. And the ‘fun’ thing isn’t fun. It’s just fluff. No one wants to do these questions more because of the rubbish around them.

The engagement comes from the thinking about the questions and their differences/similarities and ‘ahh’ moments to point out. This question set has NONE of that. Sometimes I look through my previous work and am very embarrassed. I know how long I spent looking for images I thought were cool when I should have been writing better questions!

3. Mr Barton’s New Book

Talking of better questions, I’ve been reading Reflect, Expect, Check, Explain by Craig Barton. It is, again, amazing.

I absolutely don’t mean this as an insult (hang in there, Craig, if you’re reading), but it’s full of obvious stuff. As in, you read it and think ‘Oh that’s really obvious. Why have I not being doing that?!?!’. It’s really made me reflect on my practise and how to improve what I do. I think a lot of what Craig talks about comes back to that Japanese craftswoman video. Doing things deliberately. Thinking deeply about what you do. Caring about the details. Craig is the king of this and I’m so glad he wrote this book.

4. I am making new resources…sorta

I’ve been trying to freshen up my resources. Give them a bit of a nicer look and also rewriting a lot of the questions. That process has been rather slow. I’ve done a few. You can see an updated version of my adding fractions resource here. I’m also trying to add a bit of etymology to my lessons. It’s actually been rather successful, especially thinking about links between words. And it’s interesting.

On the other hand, I’m not writing a lot of resources. I was all ready to write some circle theorems slides and then I saw the ones on mathspad which are fantastic. Lovely interactive and really clear worksheets and handouts. Why make something that would be worse?

I’m also a bit

5. Fed Up With PowerPoint

OK, so that’s not true. I still think having a PowerPoint is a way I like to plan. It’s easy to plan the narrative of what you want to do with slides. But I’m definitely removing my reliance on it. I grew up with technology and fall back far too often, even when it makes me inflexible.

For instance, I had been giving my year 8’s a Corbett Maths 5 A Day starter (which I am not having a go at and I think are ace). However it wasn’t quite working for my class. So I decided to write my questions. On the board. With a pen. Again. OBVIOUS. But I did teacher training in the age where this was a bit of a no no (downtime and all). It’s been GREAT. I’ve just been picking 5 questions of stuff from the things we’ve done this year, just written up on the board as they come in. I can be really flexible with the questions. If they struggled with something one day, I can make sure to include it the next. If they’re finding something easy I can leave it for a few lessons. I can be adaptable!

I’ve been teaching 10 years and it’s taken me all that time to realise that you don’t need to plan half a term of starters at a time. That not everything needs to be projected.

via GIPHY

What I’m doing during distance learning

Hello.

I know this is the first post in a while, but I thought I’d talk about my routines and what I’m doing during this outbreak. Maybe it will help you solidify your thoughts.

I’m going to talk about this through the prism of my year 7s.

I teach them 7 times every two weeks. So I’m advantaged by time.

I’ generally sticking to the scheme of work. For instance last week and this week have been ratio lessons, so we’ve done some work on that.

Most lessons started with a video that looks like this

A simple example-problem pair there, filmed using a visualiser. I have found the videos have worked really well for a few reasons

They’re personal. I say hello to the students and the example-problem pairs are perfectly suited to the task that follows (because I’ve created them both. They’re just print outs of the slides I make available on this website)
Everyone can watch a video. They give the students a no-excuses thing that they should have written in their book at the start of the lesson

I’ve then been following with a worksheet. These have usually been also taken from my slides (which also contain answers). I’ve found it best to make things as simple as possible. At first I was setting two tasks, now I set one. If it doesn’t take the full lesson time, so be it. This means that we’re going to have less coverage than we would have done if we’d have remained open, but I think you have to accept that things are not going to be perfect. I’d rather the basics be covered well then rushing through things.

I’ve found feedback slightly difficult. They’re uploading their work as pictures on Google Drive, but sorting through it all and marking isn’t ideal. I’m currently highlighting it and writing a little comment. I wish Google Drive had stamps! Maybe I’ll move to whole-school feedback.

I’ve tried to avoid setting too much Dr Frost (as much as I love it!) although I have used the ‘topic tests’ as check out tests to gauge their understanding.

I’ve also been trying to set work that gets them away from the computer once a week. Here’s an example.

Now this task may be a bit simple, but I think it’s worth doing because it

Get’s them off their computer
Is investigatoary.

Now, tasks like these are hard to write, but I got some nice feedback from students.

And it allowed me to pick up on their usage of language

I think it’s worthwhile setting these tasks. I’m going to set another ‘look around the house and collect stuff’ task.

This is a trying time for everyone, but it’s also a time to learn and to reflect. It’s important that we approach the time with patience and a willingness to learn.

Hope you all stay safe out there

Richard

Trigonometry

Download the PowerPoint here.

This is basically a lot of ideas knicked from Resourceaholic. Sorry @mathsjem! Quite happy with this, though. I have used this with my year 9 class this year and I think I’ve taught trig better than I ever have before.

Starts with sine. Talking about similar triangles.

Not using the calculator yet. I want them to appreciate that these are ratios, not just buttons on a calculator!

Then it moves onto two example problem pairs. I introduced the button on the calculator at this point.

Then some questions.

I went through the same process for cosine. I really spent a lot of time talking about just sine and just cosine this year. I think it really helped understanding.

Then, picking between (Don’t worry. The highlights animate in).

Then I introduced tan in the same way.

Some more practice on just tan, very much like the sin practice.

Then a mix of everything.

That was 2.5 lessons. And we didn’t even talk about finding the angle.

Trying to take the time and teach it right first time.

I think there’s possibly more to add here. I think I could have written something about answers or questions when you’re given a ratio like cosx.

Obviously there’s no problem solving AT ALL here.

There’s no mention of SOHCAHTOA. Good.

I think I’m going to write a separate trigonometry problems PowerPoint.

Why moving to teach abroad was the best thing I ever did

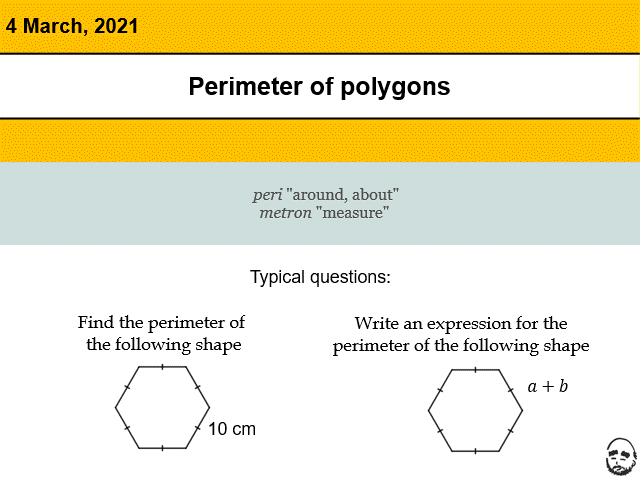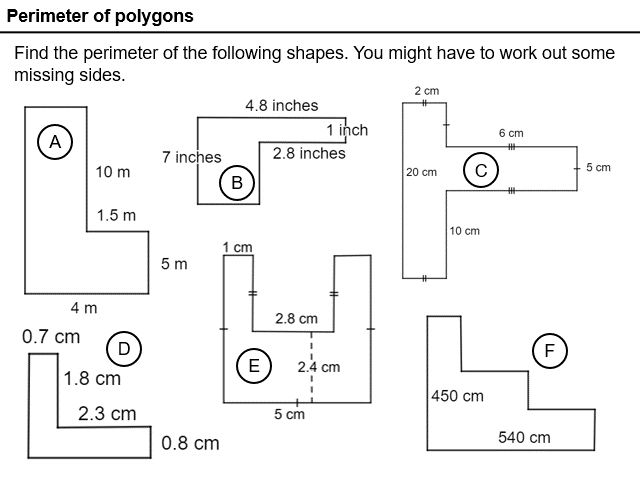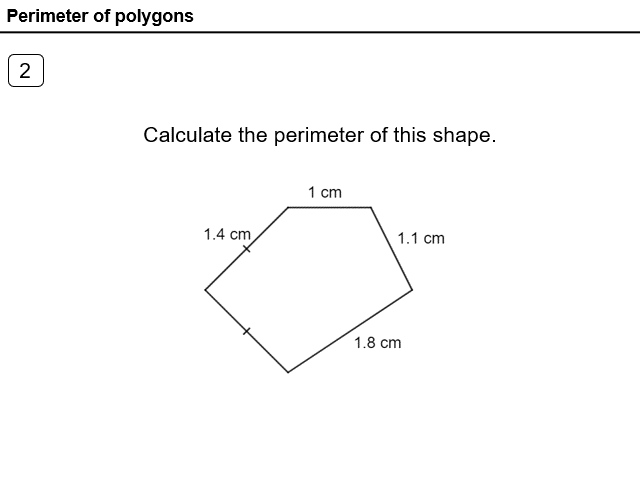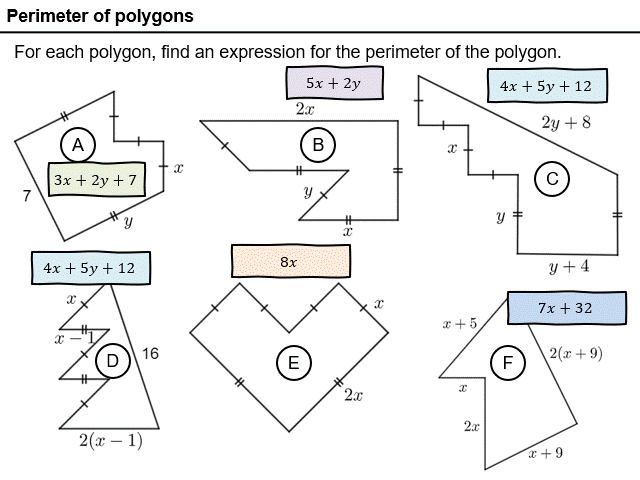
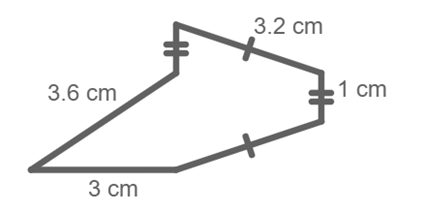
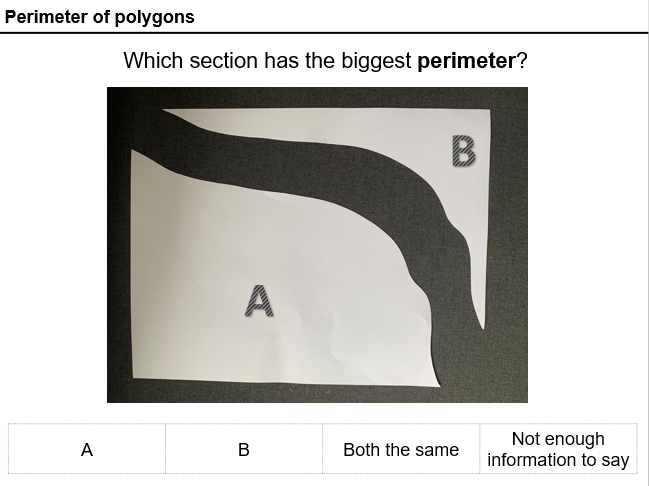
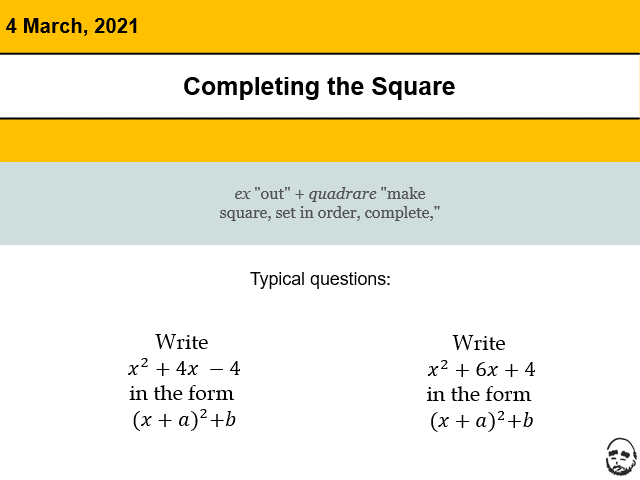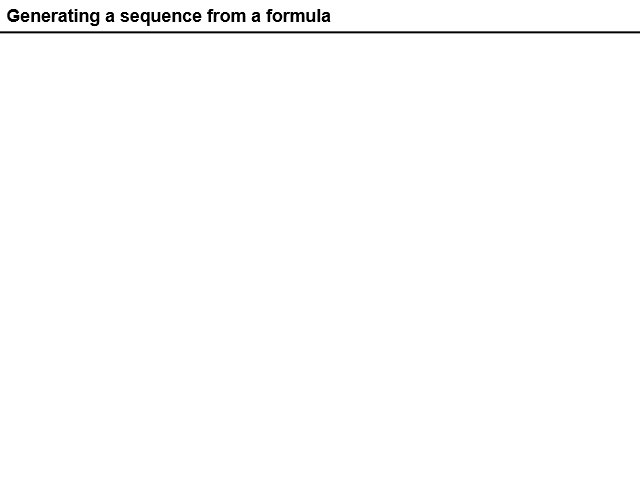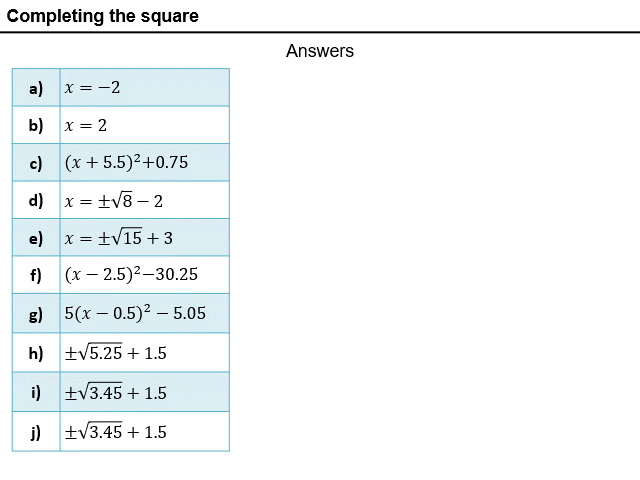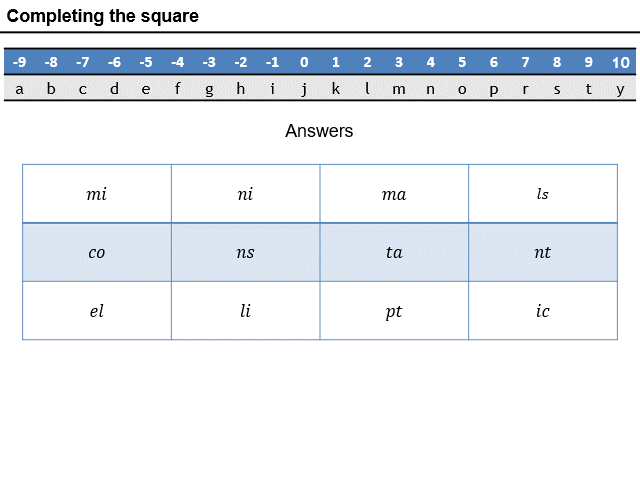
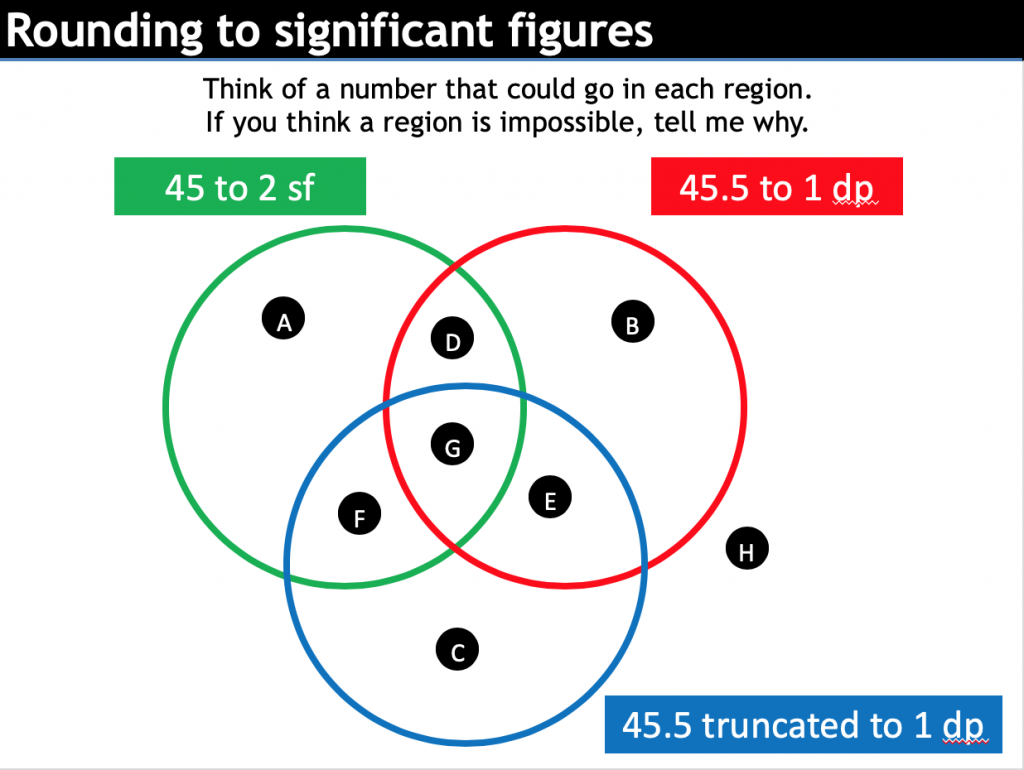
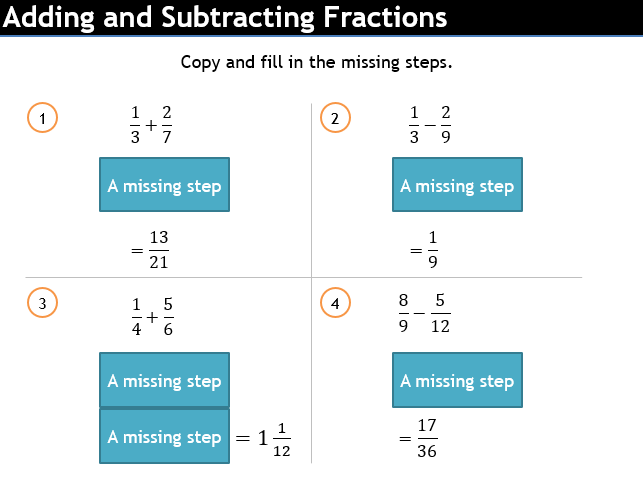
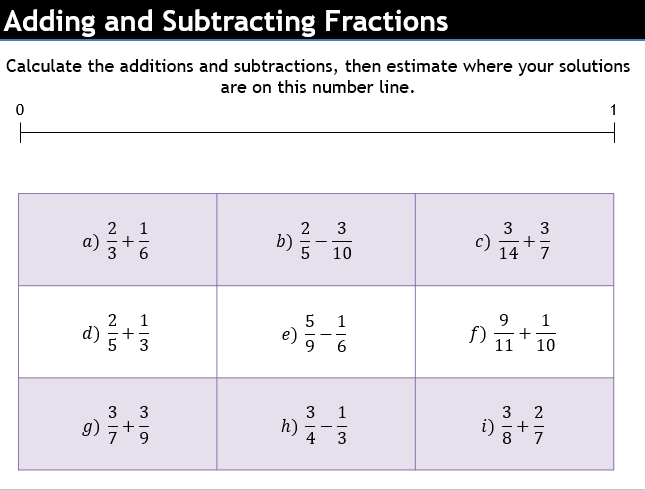
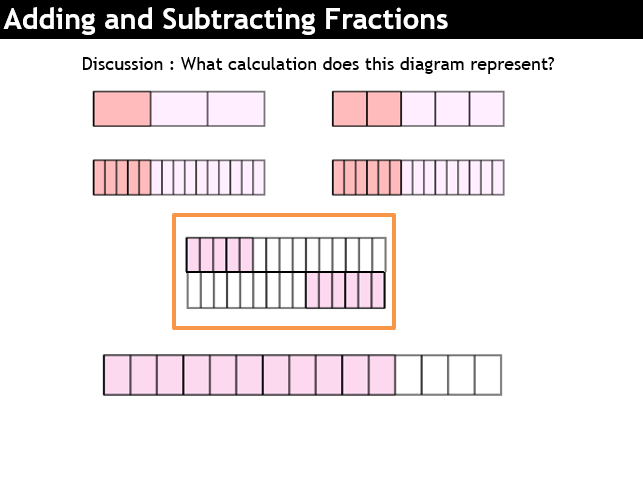
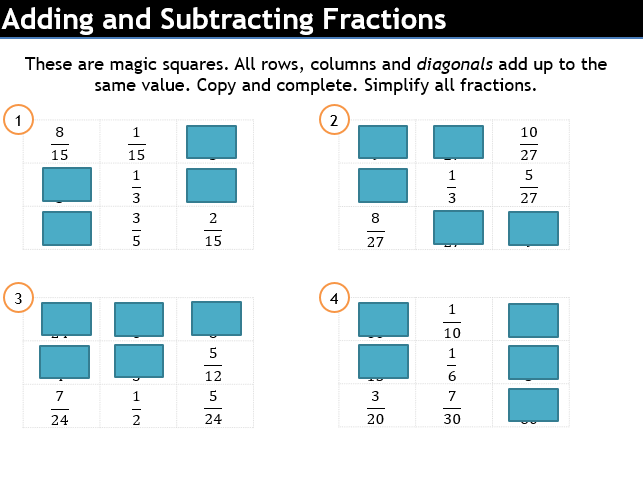
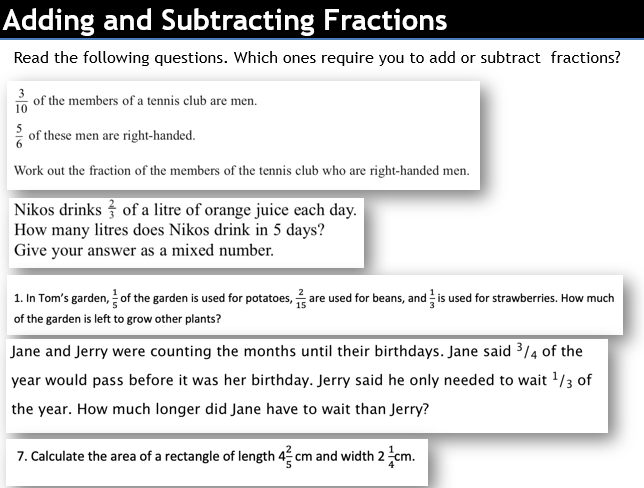
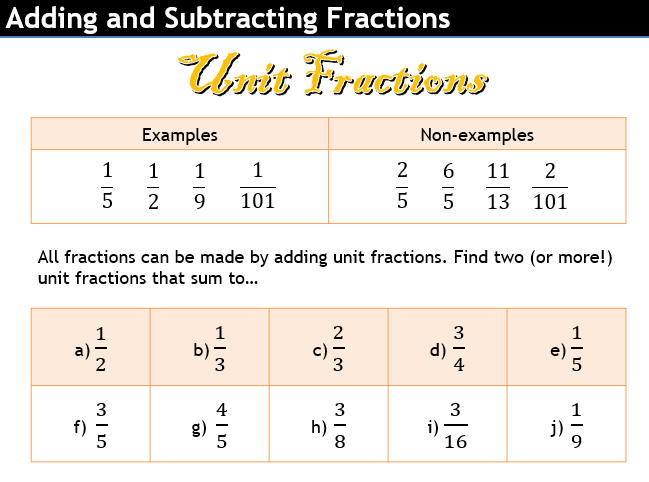
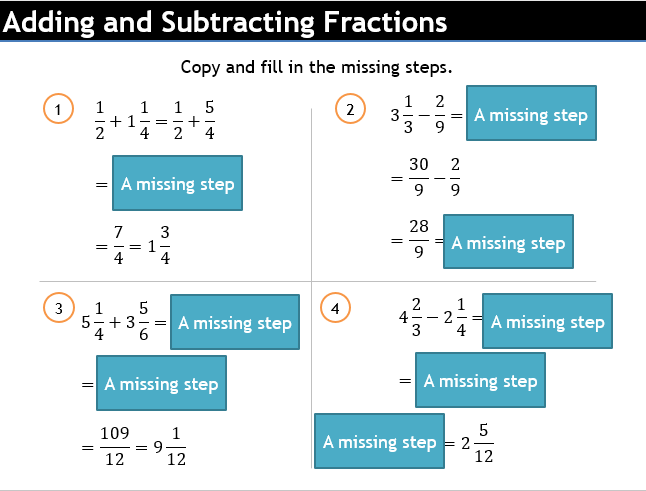
I was reading this article about teachers moving abroad. It’s a nice little read and I thought I’d add my little two cents, as this is something I’ve been thinking about recently.

In 2016 I was ready to quit teaching.

It’s easy to go through phases in teaching. Loving it, hating it, loving it again. I think a lot depends on your timetable and the structure of your day.

It had been an exhausting few years. Our school had been put into special measures a few years back and not really recovered. We were taken over by an outstanding school and went through 4 head teachers in 4 years as they scrabbled to find something that worked.

It was the behaviour that got to me eventually, though. The low level stuff that never stopped. We had a three strikes system. Three low level disruptions and you’d be removed from the lesson and put in isolation. Pupils were constantly being pulled out of my lessons. Disrupting the flow. Stopping me from actually teaching.

I blamed myself. Maybe my relationships with the children was poor. Until I did a walk around during a free period and found the number of kids being pulled out of lessons was in the double digits. Every period.

I don’t blame the school. It’s really hard to escalate these cases, and staff were trying really hard. The hours and time and commitment put into the kids at the school was miraculous. But behaviour never improved. What do you do if a kid is being removed from every lesson in a week? Exclude them? What if the behaviour continues? What if 20 kids are doing this?

I never really got upset when kids had an outburst. You can usually understand a child who punches a wall or throws a chair. But the constant cycle of low level, petty behaviours. Talking over me, throwing things. The lack of respect was what killed the joy for me.

I would go to mathsconf, or spend some time planning a great lesson, and never really get to deliver it. Then I would look into they eyes of the children who wanted to learn as we waited for SMT to remove a pupil again and just feel awful.

Then there was the UK EDUCATION STUFF.

Handing your books in to be checked. We all agreed that you couldn’t see progress in a book, but we did it anyway. We all agreed the ‘verbal feedback given’ stamp was rubbish. But it’s the done thing, you see. Eventually we got rid of the stamp, and a lot of the rules. Because the school acknowledged that it was a waste of time. But they still collected the books. To collect data. That was never used.

OFSTED came in one time and told us to be more consistent. So the school made sure that we all used the same learning objectives slide. Which was designed in the dark. Graphics stretched in the wrong ratio, a putrid green colour. Ghastly. I’m not sure that’s what OFSTED meant. I don’t think OFSTED know how their words are taken.

The worst thing was, everyone in the school wanted it to be better, and some of my colleagues where phenomenal people. Knowledgeable and committed and fantastic teachers. Dedicated, wonderful people. For some reason, it never quite came together. Which was horrible.

If all this feels like a rant, that’s because it is. I turned into a walking rant machine in my last few years in the UK!

I could have moved schools, obviously, but look on TES. It’s often the same schools in each area advertising constantly.

So I looked abroad.

It took a lot of attempts, but I ended up in a school in Thailand, and it’s been an absolutely wonderful year and a half.

I’m teaching better lessons than I ever have. I’m given the space to develop my practise and trusted. It’s made me love being a teacher again.

Our school is particularly great, to be fair. The department is fantastically organised. But the time makes the difference.

We run clubs and enrichment and we can do it because we’re not there typing up behaviour incidents 24/7.

I can take risks with my teaching. Card sorts work here! All pupils are respectful and kind and polite. I can work on a lesson and deliver it. My teaching has improved significantly because I’m not managing a room full of children, I’m teaching them. It’s great.

It’s no coincidence how much stuff has been added to this website in the last year and a half and how much better quality it is.

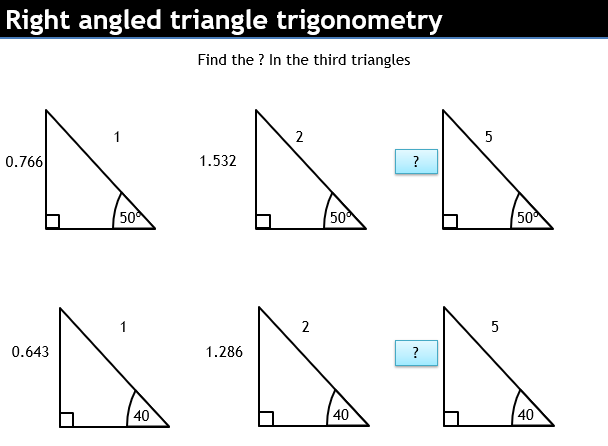
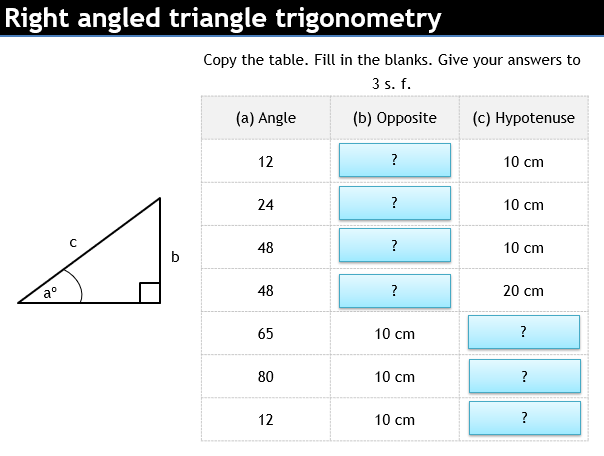
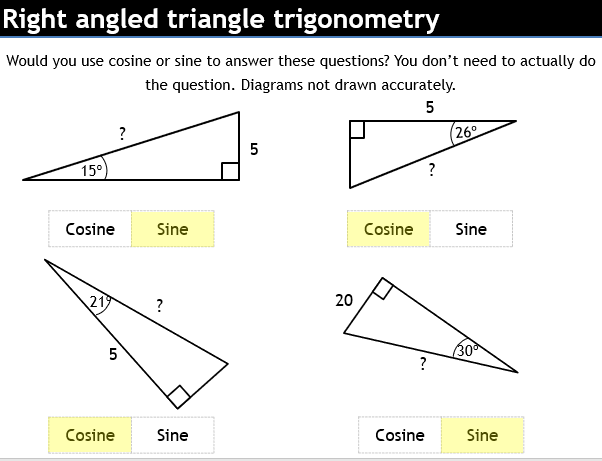
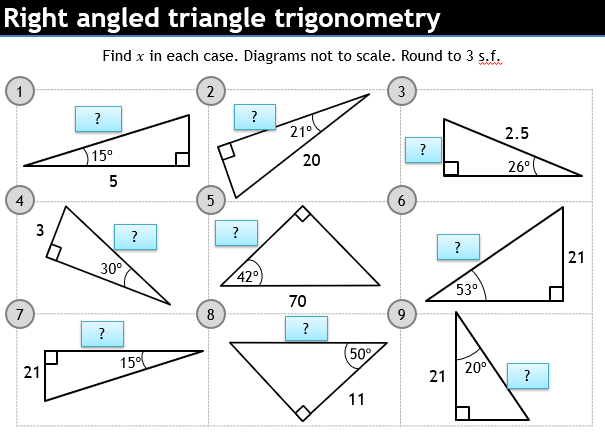
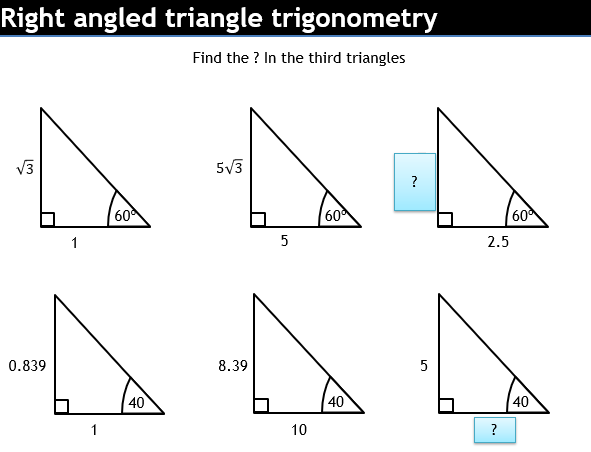
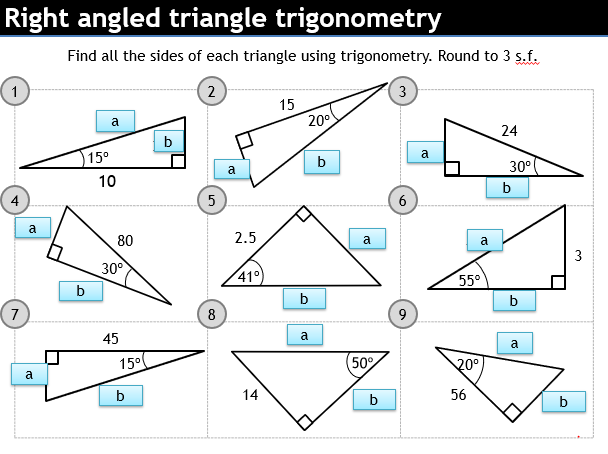
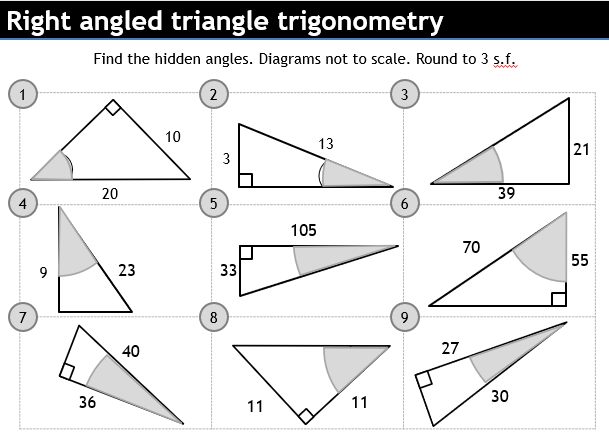
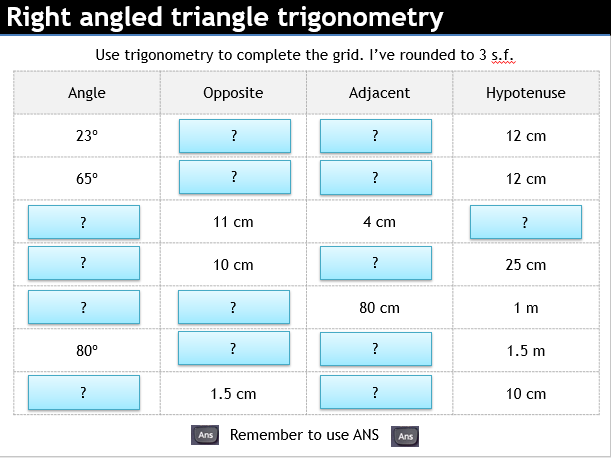
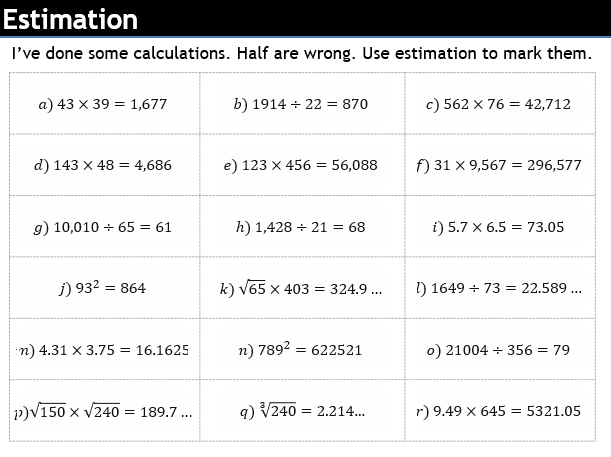
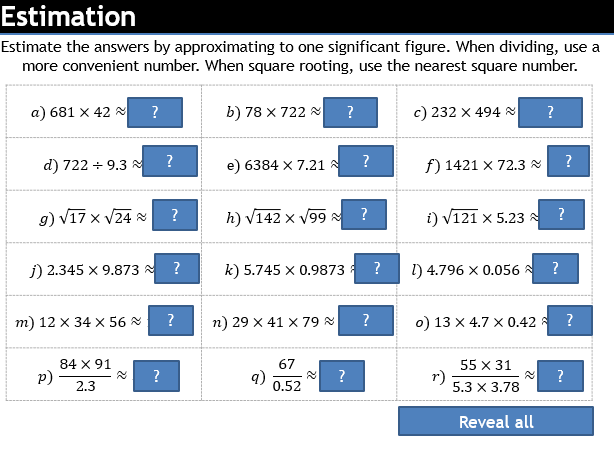
Recently I taught trigonometry to year 9 and it was joyous. We started with similar triangles. We went for understanding, not just repeating SOHCAHTOA, and it all worked because I had time to plan a good lesson and pupils who were receptive to what I was delivering.

Sometimes I have to pinch myself.

If you’re me in 2016, think about it. If you’re a bit beaten down, and feel like you could thrive if you just didn’t have to do so much rubbish that didn’t benefit the children, think about it. I would whole-heartedly recommend it.

Estimation

Download the PowerPoint here.

I created this after a discussion on Twitter.

Estimating is more complicated then I give it credit for. We have to decide the level of rounding that’s appropriate, based on the calculation being done. It’s not just as simple as rounding to 1 s.f.

I did some questions that are quite typical. Check the calculation by estimation.

Then I did some ones where we need to work it out.

And an emoji based exam question format I quite like

There’s nothing new here, nor anything really ground breaking. But it quite a bit more thorough than I have been in the past, which pleases me.

Rounding to significant figures

Download the PowerPoint here.

I didn’t want to have an example problem pair for this. Instead I went with more of a pattern spotting thing.

I think this is quite nice (it all animates in so isn’t displayed at once).

Then some fluency questions.

Then another PIXEL PUZZLE. I like these, and my ratio one is my most downloaded TES lesson.

There’s also a little venn diagram task. Mathsvenns.com by Craig Barton has got some nice ones of these.

Also all the usual gubbins. A learning check etc.

I guess I could have added some ‘real life’ stuff to this, but I didn’t want to. The football stadium holds 25,675. Round this to one significant figure etc. I find those kind of questions a bit drab.

I did, however, add this:

It provoked some discussion.

Adding fractions and mixed numbers

Download the PowerPoint here.

So, I’ve been unhappy with my adding fractions lessons for a while. They were always ‘good enough’ but not actually good. This year, I decided that good enough wasn’t good enough. I needed to finally attack them. Flesh them out, make them interesting, build in opportunity for students to really test their skills.

As I’ve gone through a lot of my older slides, I’ve removed a lot of ‘narration’. That’s true of these slides, too. Out with the clicking through written talk and animated examples, in with example problem pairs.

I thought about what I really wanted students to do. I wanted them to calculate properly, not take shortcuts. Hence :

I wanted them to get a sense of what fractions where. Not just mindless calculate, but think and interrogate their answers. Hence:

I added a little thinky thing about diagrams.

I would have added more, but I think that’s best done with something likes mathsbot.com and not with PowerPoint.

I wanted more puzzly and interesting ways to get students practicing those core skills.

I hope now you’re starting to get a sense of how big this one is!

I also wanted some good old fashioned SLOP.

I actually had these questions in the slide before. But I have tidied them up significantly. They look a lot nicer now!

What about a question where we simply have to identify WHEN we should add fractions.

I just thought of this format this week and I’m already in love with it.

I wanted to add a little mini-investigation, also.

I HAVEN’T EVEN GOT TO MIXED NUMBERS YET.

Let’s do some practice with them.

How about some practice but in a different format to change things up a bit?

What about continued fractions? Those are interesting. Maybe have a chat about those.

We could even play a game!

After I uploaded this screenshot, I noticed the bad wording at the top and edited it.

I don’t think I’ve put together a more comprehensive lesson.

There maybe too much here, but you can always skip the bits you don’t wanna do. I don’t think you could say there’s too little.

Probably won’t have another lesson for a while. It’s half term and then I don’t quite know what to make a start on next (rounding?)

Equations of perpendicular lines

Download the PowerPoint here (thanks to MrBriggs on Twitter for pointing out that I forgot to add this link in!)

This lesson went well, actually.

Work out the gradients and try and notice something.

Then there’s a little bit on making sure we can properly identify when something is a negative reciprocal.

Obviously, an example problem pair.

Then some nice questions.

Quite pleased with this one. Enjoy.

via GIPHY

Next lesson : I’m going to re-do my fractions slides and add a load of stuff in.