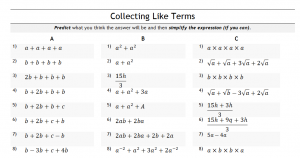
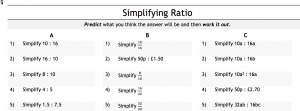
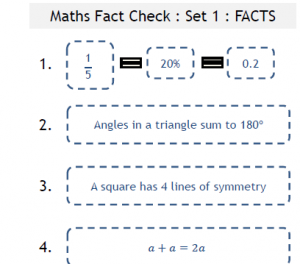
Not sure how I feel about some of the decisions here. I’ve introduced a bit of index laws towards the end of the sheet. Is this madness? I thought I would add it to reinforce the difference between simplifying powers and simplifying regular expressions. Maybe it’s too much.
As usual here’s my little justification for the first 10 questions.
- A simple one to start
- If you change the letter, it’s the same process
- You can have multiples of terms
- And it doesn’t matter where in the expression they occur
- You can have 3 terms
- And it doesn’t matter where in the expression they occur
- Introducing a negative for the first time. At the end to make it easier
- But the negative can occur anywhere! Here it actually makes you use negatives unless you collect the terms first
- Introducing terms like bc. It’s not the same as b + c
- We can do some division
Later questions cover stuff like ab being the same as ba.
I quite like the last question
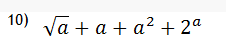
How often do we present students with expressions that can’t be simplified?
If you use it or adapt it, please comment here.